One of the toughest problems retirees face is making sure their money lasts as long as they do.
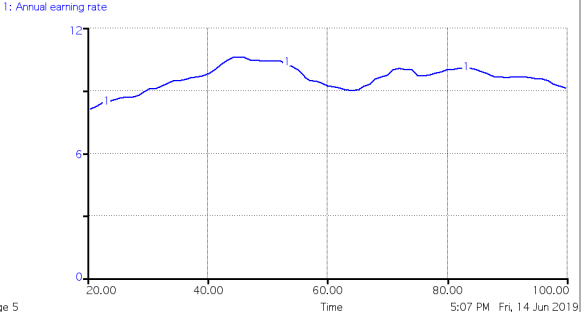
My modelling shows this is not likely to be the case for someone in Australia who enjoys continuous employment between the age of 20 and retirement at 65.
As a retiree, you also stop paying tax.
After you have been paying into your superannuation fund from the age of 20 to your retirement age of 65, you will stop making contributions and start drawing your retirement income from the superannuation fund.
Retirement incomes are tax-free in Australia. This is a very important element in retirement planning
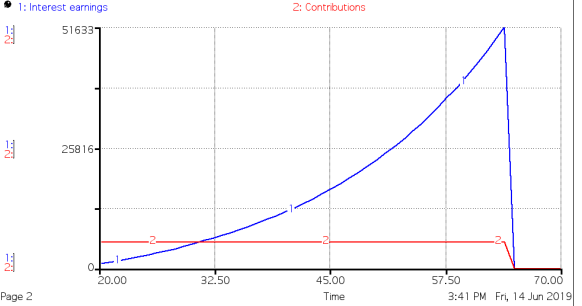
The contributions side of the model, discussed in an earlier blog, is based on an annual income of $60,000 pa, an annual interest rate of 5%, and a contribution rate of 9.5% pa.
It can be described as a very conservative model.
The model makes no allowance for inflation or cost-of-living rises to make the principles clearer.
There is now a new element to your model: the outflow Retirement income.
In this case, you have opted for a retirement income of $53,000 a year. Your take-home pay after tax on an income of $60,000 a year was $52,750.
The model also assumes that you live to be 90 years old.
In other words, you have a Retirement income equal to your take-home pay was working
The dynamics of this model now look like this:

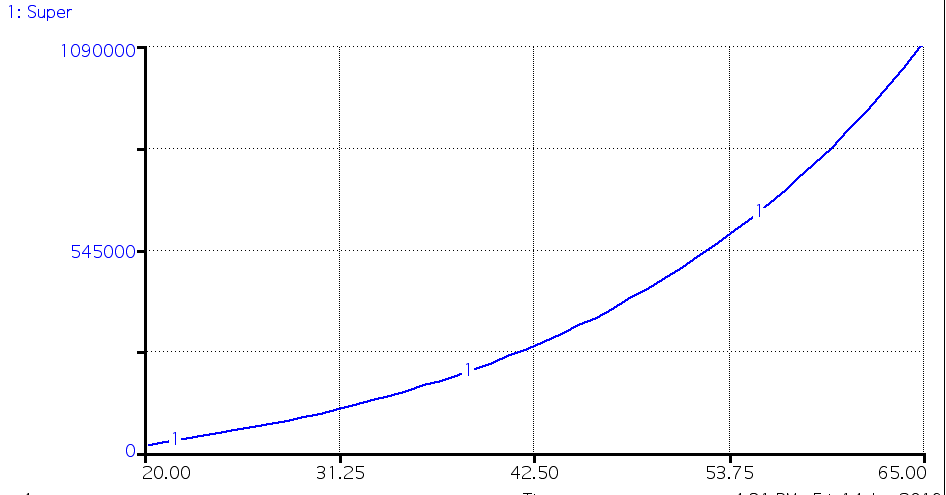
The interesting thing about this graph is that your super fund continues growing.
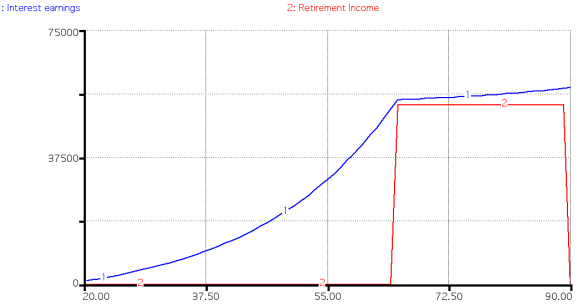
This is because your superannuation fund continues to accrue interest while you are receiving your Retirement income.
The interesting thing about these dynamics is that upon your death at the age of 90, there is still money in your super fund.
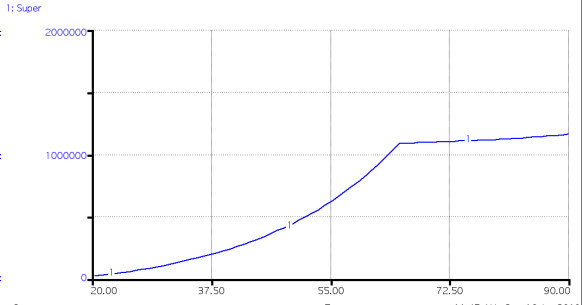
In fact there is $1.7m still in the account.
There has been discussion in the media from a number of columnists saying that superannuation funds were not designed to provide legacies for children.
But in this case, your estate will benefit by $1.7m which will be given to your dependents.
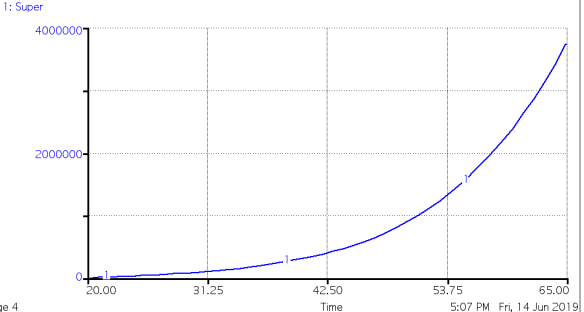
If you decide you don’t want to leave any money to your dependents, you can decide to empty your superannuation fund of the time you turn 90.
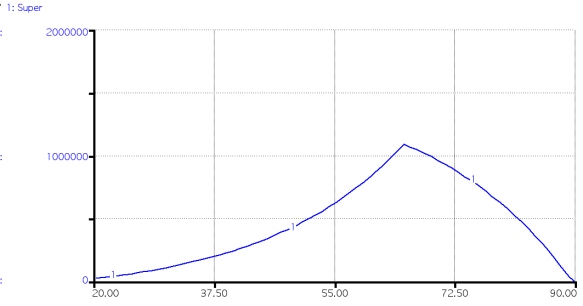
To do this you can draw a retirement income of $78,000 pa.
This is $25,000 a year more than you are earning when you were working. Enough for the occasional overseas trip.